Spanish Fencing Notation Part 5 – Strength of the Weapon
(11/3/2009)
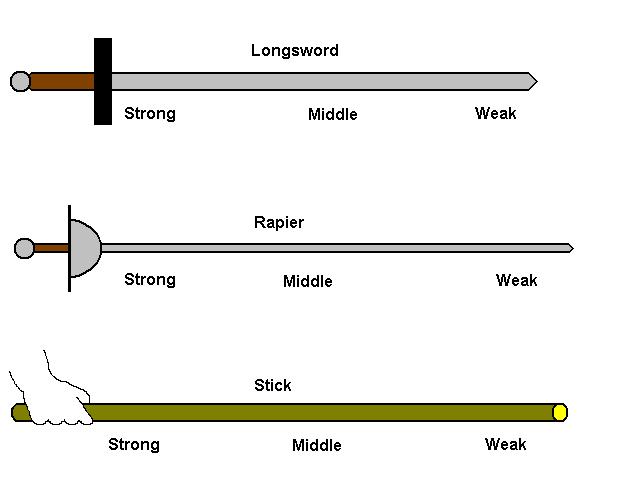
Degrees of Strength in the Weapon
The amount of physical power you can exert with a sword varies along the length of the blade. The part of the blade closest to your sword-hand has the most mechanical advantage and the further you move away from your hand the weaker you become. The Italian system typically breaks the sword down into three parts; Strong, Middle, and Weak. The same will occur for any object held in the hands whether it is a longsword, rapier, or even a stick.
While the Spanish understand this and often refer to the Strong and Weak as well, Carranza labels these parts as the Near, the Middle, and the Remote.
Carranza’s Philosophy… f.167 (1569, pub.1582)
| “Know that in the angle of the straight line, the sword has three parts. I mean that its numeration starts from the tip (as we will discuss later), and the strength increases as the numbers are multiplied until stopping in one of the centers. All this graduated quantity is divided in three equal sections with respect to the length, but they are unequal with respect to each one’s quality. You should be aware that the part next to the center’s strength is called near in this Art; the second portion, because it is between the strong and the weak and between the increase and the decrease, is the middle; and the last part is called remote.” |
~Translated by Mary Curtis
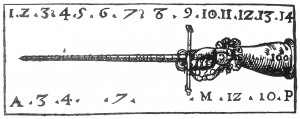
Another method of describing the transition between weaker and stronger leverage is by numbering the sword into degrees of strength. This provides us with more information when describing engagements.
Image from Carranza’s Philosophy… f. 178 (1569, pub. 1582)
Notice that the blade is numbered from one, the weakest portion, to gradually higher numbers that indicate more strength. The effect of the notation is that higher numbers defeat lower numbers which is intuitive and easy to follow. These graduations of strength in the blade can provide an instructor with a greater degree of specificity when speaking with the student.
“Engage his 4 with your 6.”
“Shift the engagement from your 3 to your 8.”
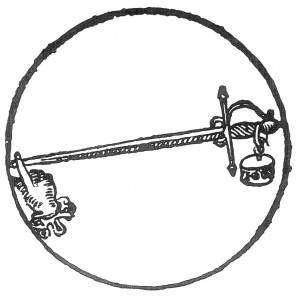
Carranza’s Leverage Demonstration
Carranza proves the mechanical advantage by applying a finger to the weakest part of a sword to demonstrate the combined effect of two different types of leverage working simultaneously.
Carranza’s Philosophy… f. 179 (1569, pub. 1582)
| “Then place the first finger of the four (called the index) on the tip of the sword at the beginning of the violent movement, and hold it firm, so that it makes two right angles with the sword. And I tell you all on behalf of the truth that even if many strong arms join together to move it from the place where the finger is, not removing it to the sides nor below, they will not move it. They will not be able to move it upward either nor make a violent movement with it upward in any way, as you all will see in this illustration that serves for the violent and natural movement.” |
~Translated by Mary Curtis
DANGER: A Bit of Physics…
To understand Carranza’s illustration, we must first define torque. When physicists talk about the linear motion of an object, we can talk about forces acting on the object. For example, if I want to move a sword in a line, I can apply a force to the sword for a thrust. These forces might be gravity pulling the object toward the earth, a fencer lifting a weapon from a table, or a lunge pushing the weapon forward.
Just as a linear force moves objects in a line, torque rotates objects around a fixed point. When we wish to rotate an object we apply force to the object to achieve torque. The length of the object (or moment arm) affects how much torque we can achieve with a given force. The further from the point of rotation you can apply your force, the greater the torque applied. Just as you can use a longer wrench to unfasten a sticky lug nut, you can also achieve greater torque by engaging the adversary’s weapon further from the axis of rotation. In plain terms, pressure on the adversary’s weapon in the weak of the blade provides you with much more strength than engagement closer to his hand.
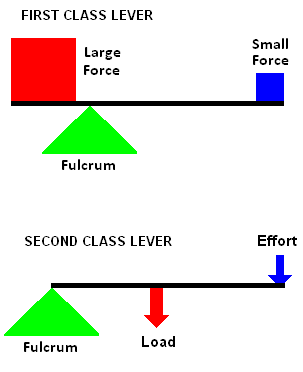
The Most Common Discussed Sword Lever
The weapon will act as a Second Class Lever when the entire arm is considered.
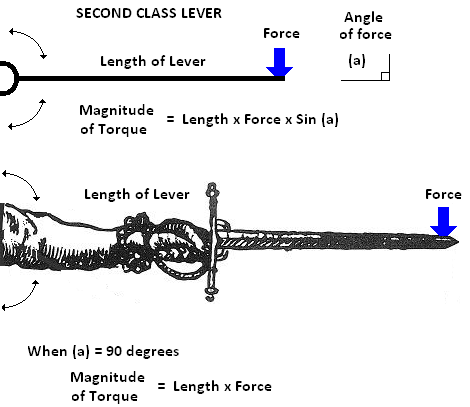
The Sword As a Second Class Lever Hinged at the Shoulder Joint. (The swordarm shown here is a reversed and edited image from Carranza's text.)
The axis of rotation might be the shoulder joint as shown here, or the elbow or wrist instead. Pushing at a 90 degree angle to the sword provides us the greatest amount of torque.
–
Without getting too deeply into mathematics, you can see that when you double the length of the lever, you also double the torque you can achieve with the same force.
- Torque = (1 foot) x (10 pounds) = 10 foot-pounds of torque
- Torque = (2 foot) x (10 pounds) = 20 foot-pounds of torque!!
- (Assuming that both are applied 90 degrees to the moment arm.)
This tells us that if we apply force further away from the shoulder in the weak of the blade we achieve greater torque than the same force applied to the strong.
Example:
You can demonstrate this in your own class by having your largest fencer grip a sword in one hand with an extended arm. Have the smaller fencer apply downward pressure to the tip with a single finger while the stronger one tries to lift the weapon from the shoulder. The large fencer will feel the torque in the shoulder because the entire extended arm and weapon becomes a Second Class Lever. If the large fencer tries to lift from the elbow or wrist, the torque will also be exerted there as well with pressure on every joint resisting the downward motion.
The Hidden Lever
There is another type of lever acting here as well that we can see by looking at Carranza’s image. In this image a heavy weight is suspended from the hilt and with a single finger we can lift it across an invisible fulcrum. What is the missing fulcrum?
Image from Carranza’s Philosophy… f. 179 (1569, pub. 1582)
Carranza’s image provides us with an example of the sword as a First Class Lever. The finger on the blade’s weak lifts the heavy weight tied to the pommel with the unseen fulcrum being the hand of the fencer holding the weapon.
The conflict between the fencer’s gripping force and pressure applied to the weapon is one of the mechanisms you might use to disarm an adversary. For example, in the previous article Carranza indicated that fingernails up is the weakest position of the hand. By applying downward pressure or a strong beat to the adversary’s weapon, you might be able to disarm your opponent. Salvator Fabris shows two possible disarms against an adversary in fourth (fingernails up) in plate 181.
Example:
You can demonstrate this in your own class by having your largest fencer grip a sword in one hand fingernails up. Have the smaller fencer apply downward pressure to the tip with a single finger. The strong fencer will feel an intense amount of pressure at the fulcrum point, and the smaller fencer will probably be able to push the tip downward. If this pressure is applied quickly and with force, it could result in a disarmament.
When Opposition Occurs Natural Defeats Violent
In a natural movement, the blade falls while in a violent movement it rises. According to the Spanish when two blades are in contact with equal degrees of strength, the fencer pushing downwards has the advantage over the fencer attempting to lift his weapon. The fencer pushing downwards can bring the weight of his upper body to bear into the engagement while the fencer resisting from below must rely solely on the muscles in the arm.
Pacheco argues that the Natural movement “…cannot be defeated by another, due to its noble nature,…” and Ettenhard echoes this.
Ettenhard in Compendium of the Foundations… p.89 (1675):
| “…and it is very clearly shown that he [don Luis Pacheco de Narvaez] said it because of the natural movement, since to it alone belongs the subjection, and in conclusion, it alone is superior to all, due to being the noblest, quickest and strongest.” |
~Translated by Mary Curtis
Changing the Relationship of the Opposition
When two blades come into opposition changing the point of contact can increase or decrease your strength in the engagement. Within the Spanish system this would be increasing or decreasing the degree of the sword.
In German longsword the practice of lifting the weapon to change a weak engagement to a stronger one is called ‘Winding’. The Spanish have a similar concept called a Movement of Increase. If both fencers start with equal engagement, Ettenhard tells us that by lifting the weapon and carrying it into the line of offense we can strengthen our engagement.
Ettenhard’s Compendium… p.119 (1675)
| “…the movement of increase, which is made in order to graduate the sword. Since for this reason more strength is acquired and increased, it is appropriately given this name but not because it is of a different type from the ones mentioned in the principles that have been defined, since this action falls (with more certainty than to any other) to the mixed movement aligning lateral and violent.” |
~Translated by Mary Curtis
In contrast, moving from a strong engagement to a weaker one is called a Movement of Decrease. If both fencers start with equal engagement, then by lowering the weapon and moving away from the line of offense we weaken our engagement.
Ettenhard’s Compendium… p.119 (1675)
| “The movement of decrease is the one that is made in order to reduce the strength, disgraduating the sword, whose action belongs legitimately to the mixed movement of offline lateral and natural…” |
~Translated by Mary Curtis
This again reinforces that the high line provides the greatest advantage and that engagement from below is considered weaker by the Spanish tradition.
A Riddle of Inertia, Momentum, and Energy
It might be best to consider a fencer’s riddle and then examine some physics to provide us with an answer.
Riddle: How can you parry a greatsword with a dagger?
DANGER: A Bit More Physics…
Inertia is an object’s resistance to a change in the state of its motion. Any object that is not moving requires force to accelerate it. For example, to swing a cut you will apply a force to the weapon to accelerate it towards the adversary’s head. If we ignore rotational motion in this discussion, the required force is defined as:
Force = (mass of sword) x (acceleration)
In order to decelerate or stop a moving object you have to apply the same type of force.
Momentum is defined as the product of velocity and mass which does not provide us much perspective into its effect in swordplay, but it is useful for determining behavior during collisions because momentum is conserved. That means that a heavy object moving very quickly (like a greatsword) that is forcibly stopped by a smaller one will transfer momentum to the smaller object.
Momentum = (mass of sword) x (sword velocity)
Kinetic Energy tells us how much energy is delivered with an attack. The most important element in delivering a powerful attack is the velocity as shown here:
Kinetic Energy = 1/2 x (mass of sword) x (sword velocity) x (sword velocity)
By plugging in some numbers we can see how this might turn out. First, we can weight the two equally as shown here:
- Mass = 2
- Velocity = 2
Kinetic Energy = 1/2 x (2) x (2) x (2) = 4
If we double the mass we achieve an increase in energy as shown here:
- Mass = 4
- Velocity = 2
Kinetic Energy = 1/2 x (4) x (2) x (2) =8
If we instead double the velocity we achieve a much more powerful attack:
- Mass = 2
- Velocity = 4
Kinetic Energy = 1/2 x (2) x (4) x (4) =16
Carranza Answers the Riddle
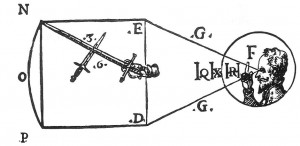
In Carranza’s Philosophy on folio 180 we see an odd figure with a dagger, a sword, a hand, and a head surrounded by geometric figures. (This is one of only six figures in the treatise.)
If you close one eye and lift your index finger, holding it away from your face like the man in the image, it obscures only a very tiny portion of your sight. As you bring your finger back towards your eye (the source of your sight) it becomes larger and obscures more. If your finger is close enough to the origin, it can block out your entire view. What this tells us is that even a tiny finger can obscure a large object when it intercepts the vision at the beginning.
Carranza uses this as an analogy to tell us that in the same way, even a dagger can parry a powerful greatsword if it intercepts the cut close to the beginning of the attack. (Looking at the image above you can imagine intercepting the attack where it begins at the point labeled N.) Because the velocity is close to zero it is easy to halt (Inertia), the Momentum is low, and the Kinetic Energy delivered at the beginning of the cut is also very low.
If you intercept the same cut at the point labeled O, the velocity of the attack has increased and your parry can easily fail.
Riddle: How can you parry a greatsword with a dagger?
Carranza’s Philosophy… f.180 (1675)
| “These movements are weak in all their beginnings,… “ |
~Translated by Mary Curtis
Answer: Parry the attack when the velocity is zero or very low.
Conclusion
I have used the physics described by Isaac Newton’s Philosophiæ Naturalis Principia Mathematica to explain some of the different aspects of how a sword functions but this text wasn’t published until 1687. The equation for kinetic energy was not discovered until 1829 by a French mathematician named Gustave Coriolis. Even considering that the fencing masters of Spain used the physics and motion described by classical authors like Aristotle, they knew qualitatively how weapons moved in combat which provides their work with a high level of sophistication.